|
C. Grosche II.lnstitut f  r Theoretische Physik Universit r Theoretische Physik Universit t Hamburg, Luruper Chaussee 14922761 Hamburg, Germany t Hamburg, Luruper Chaussee 14922761 Hamburg, Germany
G. S. Pogosyan, A. N. Sissakian Bogoliubov Laboratory of Theoretical Physics Joint Institute for Nuclear Research 141980 Dubna, Moscow Region, Russia
This paper is the third in a series, in which we try to generalize the notion of superintegrable potentials, as known from the flat space, to the case of spaces of constant negative curvature. Path integral approach to superintegrable potentials on the two-dimensional hyperboloid is presented. We find five potentials of the sought type, which possess three functionally independent integrals of motion (observables), and in each case we present the appropriate path integral formulation. We list in the soluble cases the path integral solutions explicitly in terms of the propagators, the Green functions, and the spectral expansions in the wave functions. The coordinate systems on the two-dimensional hyperboloid are discussed in detail. The St Настоящая работа является третьей из серии работ, в которой обобщено понятие суперинтегрируемых потенциалов, известных для плоского пространства на случай пространств постоянной отрицательной кривизны. Сформулирован метод континуального интегрирования для суперинтегрируемых потенциалов на двумерном гиперболоиде. Найдены пять потенциалов искомого типа, которые содержат три функционально независимых интеграла движения (наблюдаемых), и в каждом случае выписаны соответствующие интегралы по траекториям. Описаны все случаи, где с помощью метода континуального интегрирования возможно решение в явном виде на языке пропагаторов, функций Грина и спектральных разложений по волновым функциям. Обсуждаются все возможные ортогональные системы координат на двумерном гиперболоиде. Для каждой из систем координат построены оператор Шредингера и матрица Штеккеля, приведены соответсвующие интегралы движения. Особое внимание уделено обобщению гармонического осциллятора Хиггса, и задачи Кеплера - Кулона. Оставшиеся три потенциала являются аналогами потенциала Холта и центробежного, а последняя модель соответсвует в пределе плоского пространства линейному потенциалу. Full text in PDF (2.036.662) |
 Home
Home
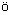 dinger operator, the general form of the potential, which must be separable, and relevant observables are constructed for each coordinate system. A special care is taken of the proper generalization of the harmonic oscillator on the hyperboloid, i.e., the Higgs-oscillator, and the Kepler - Coulomb problem. The three remaining potentials are analogues of the Holt potential, the centrifugal potential, and the last one is the potential which is linear in the flat space limit.
dinger operator, the general form of the potential, which must be separable, and relevant observables are constructed for each coordinate system. A special care is taken of the proper generalization of the harmonic oscillator on the hyperboloid, i.e., the Higgs-oscillator, and the Kepler - Coulomb problem. The three remaining potentials are analogues of the Holt potential, the centrifugal potential, and the last one is the potential which is linear in the flat space limit.