|
The Compression Modes in Atomic Nuclei and Their Relevance
for the Nuclear Equation of State
Colò G.
Accurate assessment of the value of the incompressibility coefficient, K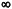 ,
of symmetric nuclear matter, which is directly related to the curvature of
the equation of state (EOS), is needed to extend our knowledge of the EOS
in the vicinity of the saturation point. We review the current status of K ,
of symmetric nuclear matter, which is directly related to the curvature of
the equation of state (EOS), is needed to extend our knowledge of the EOS
in the vicinity of the saturation point. We review the current status of K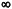 ,
as determined from experimental data on isoscalar giant monopole and dipole
resonances (compression modes) in nuclei, by employing the microscopic theory
based on the Random Phase Approximation (RPA). The importance of full
self-consistent calculations is emphasized. In recent years, the
comparison between RPA calculations based on either nonrelativistic
effective interactions, or relativistic Lagrangians, has been pursued
in great detail. It has been pointed out that these two types of models
embed different ansatz for the density dependence of the symmetry energy.
This fact has consequences on the extraction of the nuclear incompressibility,
as it is discussed. The comparison with other ways of extracting
K ,
as determined from experimental data on isoscalar giant monopole and dipole
resonances (compression modes) in nuclei, by employing the microscopic theory
based on the Random Phase Approximation (RPA). The importance of full
self-consistent calculations is emphasized. In recent years, the
comparison between RPA calculations based on either nonrelativistic
effective interactions, or relativistic Lagrangians, has been pursued
in great detail. It has been pointed out that these two types of models
embed different ansatz for the density dependence of the symmetry energy.
This fact has consequences on the extraction of the nuclear incompressibility,
as it is discussed. The comparison with other ways of extracting
K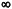 from experimental data is highlighted. from experimental data is highlighted.
PDF (600 Kb)
|
